Question

Which equation justifies why ten to the one third power equals the cube root of ten?

ten to the one third power all raised to the third power equals ten to the one third plus three power equals ten

ten to the one third power all raised to the third power equals ten to the one third times three power equals ten

ten to the one third power all raised to the third power equals ten to the three minus one third power equals ten

ten to the one third power all raised to the third power equals ten to the one third minus three power equals ten

Answer 1

Answer: The answer would be (10^1/3)³ = 10 ^(1/3×3) =10

Explanation:

I took the test on FLVS

Answer 2

For this case we must find the justification of:

`10 ^ {\frac {1} {3}} = \sqrt [3] {10}`

By definition of properties of powers and roots we have to meet:

`a ^ {\frac {m} {n}} = \sqrt [n] {a ^ m}`

So, if we have:

`\sqrt [3] {10} = \ \sqrt [3] {10 ^ 1} = 10 ^ {\frac {1} {3}}`

Other property states that:

`(a ^ n) ^ m = a ^ {n * m}`

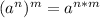
So, the expression: "Ten to the one third power all raised to the third power" is represented as:

`(10 ^{\frac {1} {3}}) ^ 3 = 10 ^ {\frac {3*1} {3}} = 10 ^ 1 = 10`

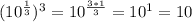
ANswer;

Option B