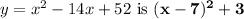Option c
Answer:
The vertex form for
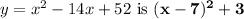
Solution:
The standard form of equations is given as
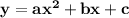
The general representation of vertex form is
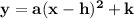 where (h,k) is the vertex of the parabola.
where (h,k) is the vertex of the parabola.
The "a" in the vertex form is the same "a" as in
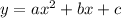 (standard form)
(standard form)
The value of “a” is same in both standard and vertex form.
In order to represent the given expression into vertex form follow the below steps:
From question, Given equation is
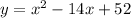 .This equation can be rewritten as
.This equation can be rewritten as
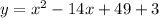
Where “52” has been rewritten as 49 + 3.
Now, 14x can be written as 2(7x) and 49 can be written as
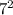 . Hence the above equation becomes,
. Hence the above equation becomes,
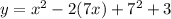
The first three terms of above equation is of the form
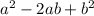 , where a = 1 and b = 7
, where a = 1 and b = 7
We know that
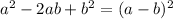
Hence
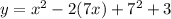 becomes,
becomes,
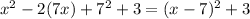
Now
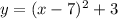 is of the form
is of the form

Where by comparing we get, a = 1 and h = 7 and k = 3
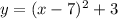
Hence the vertex form of