Question

If an integer n has three unique prime divisors, then it follows that the largest prime divisor of n is less than or equal to cube root of n. True or False?

Answer 1

False

Explanation:

Lets call the three prime divisors of n p, q, and r, being r the largest, we know:

`n=q * p * r`

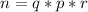
Now, if

`q * p < r`

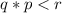
then

`n < r * r = r^2`

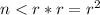
So:

`√(n) < √(r^2) = r`

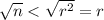
Also, for every natural greater than one, we know:

`\sqrt[3]{n}<√(n)`

so

`\sqrt[3]{n}<√(n) < r`

from which:

`\sqrt[3]{n} < r`

So, we see, this means the preposition is false, we can find a particular counterexample:

q=2

p=3

p*q = 6

We need to choose a prime greater than 6

r=7

n= 2 * 3 *7 = 42

`\sqrt[3]{42} = 3.4760 < 7`