Question

PLEASE HELP!!! I NEED WORK SHOWN ILL LOVE YOU FOREVER

Answer 1

`(x^(2)+2x+5 )/(4) +(10)/(x+4)`

OR

`(x^(2)+5 )/(4)+(x)/(2)+(5)/(x+2)`

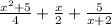
Explanation:

PART A:

At the bottom, the x can be interpreted as
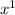`x^(1)` and when dividing exponents, you subtract them, so every exponent at the top will be losing a value. ((Also, I forgot to put it, but, x^2 should also be /4 ----> x^2/4

You can actually also simplify the numbers (that are divisible by 4) but the equation will look a bit different.

PART B: Basically, you are undoing everything you just did...

Remember that multiplying exponents is the equivalent of adding numbers.