Question

A curved road has a radius of 120 m and a cant angle of 48 degrees. What is the maximum speed to stay on the curve in the absence of friction? a. 16 m/s

b. 28 m/s
c. 24 m/s
d. 36 m/s

Answer 1

Maximum speed, v = 36 m/s

Step-by-step explanation:

Given that,

The radius of the curved road, r = 120 m

Road is at an angle of 48 degrees. We need to find the maximum speed of stay on the curve in the absence of friction. On a banked curve, the angle at which it is cant is given by :

`tan\theta=(v^2)/(rg)`

g is the acceleration due to gravity

`v=√(rg\ tan\theta)`

`v=√(120* 9.8* \ tan(48))`

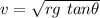
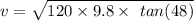
v = 36.13 m/s

or

v = 36 m/s

So, the maximum speed to stay on the curve in the absence of friction is 36 m/s. Hence, this is the required solution.