Answer:
There are 795 combinations.
Explanation:
The number of ways or combinations in which we can select k element from a group of n elements is given by:
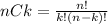
So, if Miriam want to choose 3 movies with at least two comedies, she have two options: Choose 2 comedies and 1 foreign film or choose 3 comedies.
Then, the number of combinations for every case are:
1. Choose 2 Comedies from the 10 and choose 1 foreign film from 15. This is calculated as:
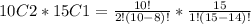
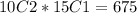
2. Choose 3 Comedies from the 10. This is calculated as:
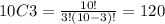
Therefore, there are 795 combinations and it is calculated as:
675 + 120 = 795