Answer:
Torque at input shaft will be 176.8695 N-m
Step-by-step explanation:
We have given input power

Angular speed = 2300 rpm
For converting rpm to rad/sec we have multiply with
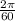
So
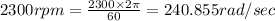
We have to find torque
We know that power is given by
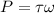 , here
, here
 is torque and
is torque and
 is angular speed
is angular speed
So
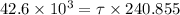
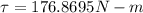
So torque at input shaft will be 176.8695 N-m