Answer:
The total profit is 612.5
Step-by-step explanation:
First we need to find the profit maximizing quantity. Since the monopolist faces the entire demand his profit (
 )equation would be
)equation would be
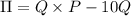
where PxQ is his revenue and 10Q is his total cost.
We can replace P in the above equation from the equation demand
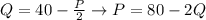
Then
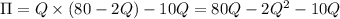
taking derivatives with respect to Q

then Q=17.5 and P=45.
The total profit is then 612.5