Question

The value of an investment A (in dollars) after t years is given by the function A(t) = A0ekt. If it takes 10 years for an investment of $1,000 to triple, how many years will it take for the investment to be $9,000? Simplify your answer completely.

Answer 1

20 years.

Explanation:

We have been given a formula
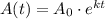`A(t)=A_0\cdot e^(kt)`, which represents the value of an investment A (in dollars) after t years.

Substitute the given values:

`\$3,000=\$1,000\cdot e^(k*10)`

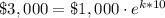
Let us solve for k.

`(\$3,000)/(\$1,000)=(\$1,000\cdot e^(k*10))/(\$1,000)`

`3=e^(k*10)`

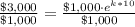
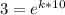
Take natural log of both sides:

`\text{ln}(3)=\text{ln}(e^(k*10))`

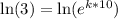
Using property
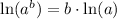`\text{ln}(a^b)=b\cdot \text{ln}(a)`, we will get:

`\text{ln}(3)=10k\cdot\text{ln}(e)`

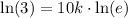
We know that
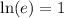`\text{ln}(e)=1`, so

`\text{ln}(3)=10k\cdot 1`

`\text{ln}(3)=10k`

`\frac{\text{ln}(3)}{10}=(10k)/(10)`

`\frac{\text{ln}(3)}{10}=k`

`\$9,000=\$1,000\cdot e^{\frac{\text{ln}(3)}{10}*t}`

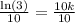
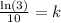
Dividing both sides by 1000, we will get:

`9=e^{\frac{\text{ln}(3)}{10}*t}`

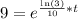
Take natural log of both sides:

`\text{ln}(9)=\text{ln}(e^{\frac{\text{ln}(3)}{10}*t)`

`\text{ln}(9)=\frac{\text{ln}(3)}{10}*t\cdot\text{ln}(e)`

`\text{ln}(9)=\frac{\text{ln}(3)}{10}*t\cdot1`

`10*\text{ln}(9)=10*\frac{\text{ln}(3)}{10}*t`

`10\text{ln}(9)=\text{ln}(3)*t`

`10\text{ln}(3^2)=\text{ln}(3)*t`

`2\cdot 10\text{ln}(3)=\text{ln}(3)*t`

`20\text{ln}(3)=\text{ln}(3)*t`

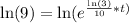
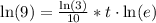
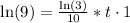
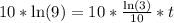
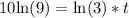
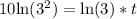
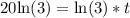
Divide both sides by
`\text{ln}(3)`:

`\frac{20\text{ln}(3)}{\text{ln}(3)}=\frac{\text{ln}(3)*t}{\text{ln}(3)}`

`20=t`

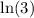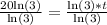
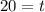
Therefore, it will take 20 years for the investment to be $9,000.