Answer:
15.
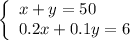
16. 10 ml of 20% saline and 40 ml of 10% saline
Explanation:
A chemist takes x ml of 20% saline and y ml of 10% saline. In total, he takes
x + y ml that is 50 ml, so
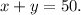
There are
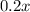ml of salt in x ml of 20% saline and
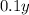ml of salt in 10% saline. There are
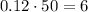ml of salt in 50 ml of 12% saline. Thus,
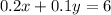
15. We get the system of two equations:
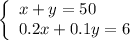
16. Solve this system. From the first equation:
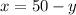
Substitute it into the second equation:
