Given :
A curve : 3x² - 2xy + y² = 11
To Find :
The slope of the line tangent to the curve 3x^2-2xy+y^2=11 at the point (1, -2).
Solution :
Differentiating given curve w.r.t x is :
6x - ( 2y + 2xy' ) + 2yy' = 11
Putting the point ( 1, -2) in above equation, we get :
6 - ( 2(-2) + 2y' ) - 4y' = 11
6 - ( -4 + 2y' ) - 4y' = 11
10 - 6y' = 11
6y' = -1
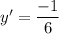
Therefore, the slope of the line tangent to the given point is
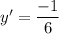 .
.