Step-by-step explanation:
Force applied on the gas will be as follows.
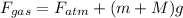
As, F = pressure × area. Hence, calculate the forces as follows.
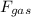 = pressure × area
= pressure × area
=
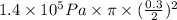
=
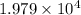 N
N
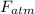 = pressure × area
= pressure × area
=
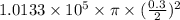
=
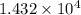 N
N
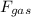 -
-
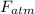 =
=
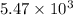 N
N
Substituting the calculated values into the above formula as follows.
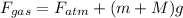
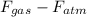 = (m + M) g
= (m + M) g
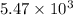 N =
N =

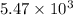 N =
N =
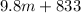
m = 472.76 kg
Thus, we can conclude that the mass is 472.76 kg.