Question

Given that events "A" and "B" are independent, P(A)= 0.80 and P(A and B) = 0.24, what is P (B)?

Group of answer choices

0.104

0.192

0.56

0.30


A weather forecaster predicts that their is 50% chance of rain on Saturday and a 40% chance of rain on Sunday. If these probabilities are correct, what is the probability that it will rain both days?

Group of answer choices

20%

45%

10%

90%


A card is randomly drawn from a shuffled deck of cards and NOT REPLACED. A second card is drawn from the remaining shuffled cards. What is the approximate probability that both cards are RED?


49%

50%

24.5%

25%

Answer 1

1. 0.30

2. 20%

3. is not 24.5%

Explanation:

Answer 2

Answer: The correct options are

(1) (D) 0.30

(2) (A) 20%

(3) (C) 24.5%.

Step-by-step explanation: We are given to answer all the following three questions.

(1) Given that A and B are independent events, where

`P(A)=0.80,~~P(A\cap B)=0.24,~~~P(B)=?`

We know that

if S and T are independent events, then

`P(S\cap T)=P(S)* P(T).`

Therefore, we get

`P(A\cap B)=P(A)\cap P(B)\\\\\Rightarrow 0.24=0.80* P(B)\\\\\Rightarrow P(B)=(0.24)/(0.80)\\\\\Rightarrow P(B)=0.30.`

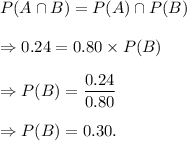
Option (D) is CORRECT.

(2) Given that a weather forecaster predicts that their is 50% chance of rain on Saturday and a 40% chance of rain on Sunday.

We are to find the probability that it will rain both days.

Let X and Y represents the probabilities that it will rain on Saturday and Sunday respectively.

Then, we have

`P(X)=50\%=(50)/(100)=(1)/(2),\\\\\\P(Y)=40\%=(40)/(100)=(2)/(5).`

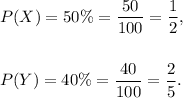
Since X and Y are independent of each other, so the probability that it will rain both days is

`P(X\cap Y)=P(X)* P(Y)=(1)/(2)*(2)/(5)=(1)/(5)*100\%=20\%.`

Option (A) is CORRECT.

(3) Given that a card is randomly drawn from a shuffled deck of cards and NOT REPLACED. A second card is drawn from the remaining shuffled cards.

We are to find the probability that both cards are RED.

Since there are 26 red cards in a pack of 52 cards, so the probability of drawing first red card is

`p_1=(26)/(52)=(1)/(2).`

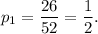
Without replacement, the probability of drawing second red card will be

`p_2=(25)/(51).`

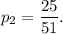
Therefore, the probability that both cards are red is

`p=p_1* p_2=(1)/(2)*(25)/(51)=(25)/(102)=0.245*100\%=24.5\%.`

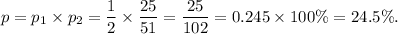
Option (C) is CORRECT.

Thus, (D), (A) and (C) are correct options.