Question

There has been a great deal of controversy over the last several years regarding what types of surveillance are appropriate to prevent terrorism. Suppose a particular surveillance system has a 98% chance of correctly identifying a future terrorist and a 99.9% chance of correctly identifying someone who is not a future terrorist. If there are 1,000 future terrorists in a population of 300 million, and one of these 300 million is randomly selected, scrutinized by the system, and identified as a future terrorist, what is the probability that he/she actually is a future terrorist? (Round your answer to six decimal places.)

Answer 1

Given that a person was identified as a future terrorist, there is a 2.8544% probability that he/she actually is a future terrorist.

Explanation:

There are 1000 future terrorists in a population of 300,000,000. So the probability that a randomly selected person in this population is a terrorist is:

`P = (1,000)/(300,000,000) = 0.000003 = 0.0003%`

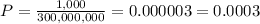
So, we have these following probabilities:

A 99.9997% probability that a randomly chosen person is not a terrorist.

A 0.0003% probability that a randomly chosen person is a terrorist.

A 98% probability that a future terrorist is correctly identified

A 99.9% chance of correctly identifying someone who is not a future terrorist. This also means that there is a 0.01% probability of someone who is not a terrorist being identified as one.

This can be formulated as the following problem:

What is the probability of B happening, knowing that A has happened.

It can be calculated by the following formula

`P = (P(B).P(A/B))/(P(A))`

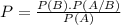
Where P(B) is the probability of B happening, P(A/B) is the probability of A happening knowing that B happened and P(A) is the probability of A happening.

Here we have:

What is the probability that the person is a terrorist, given that she was identified as a terrorist.

P(B) is the probability that the person is a terrorist. So
`P(B) = 0.000003`

P(A/B) is the probability that the person was identified as a terrorist, given that she is a terrorist. The problem states that the system has a 98% chance of correctly identifying a future terrorist, so
`P(A/B) = 0.98`

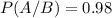
P(A) is the probability of a person being a identified as a terrorist. So

`P(A) = P_(1) + P_(2)`

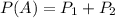
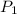`P_(1)` is the probability that a person is a terrorist and was identified as one. So:

`P_(1) = 0.000003*0.98 = 0.00000294`

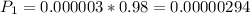
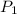`P_(1)` is the probability that a person is not a terrorist and, but was identified as one. So:

`P_(2) = 0.999997*0.0001 = 0.0000999997`

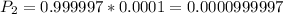
So

`P(A) = P_(1) + P_(2) = 0.00000294 + 0.0000999997 = 0.000103`

The answer is:

`P = (P(B).P(A/B))/(P(A)) = (0.000003*0.98)/(0.000103) = 0.028544`

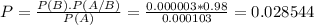
Given that a person was identified as a future terrorist, there is a 2.8544% probability that he/she actually is a future terrorist.