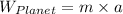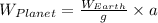Question

Consider an object with weight on the Earth W_earth. The gravity of the Earth is g. If it is moved to another planet with gravity a, how much will be its weight? Note: you don't need to put a number for variables, M, W, a, g

Answer 1

`W_(Planet) = (W_(Earth))/(g)* a`

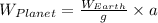
Step-by-step explanation:

We know that weight of an object on Earth is,

`W_(Earth) = m* g`

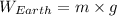
Thus,

`m = (W_(Earth))/(g)`

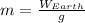
where,

m = mass of an object, which is constant and is independent of gravity

g = acceleration due to gravity on Earth

On the new planet, gravity = a

Thus the weight of the object on the new planet will be

`W_(Planet) = m* a`

`W_(Planet) = (W_(Earth))/(g)* a`