Question

A street light is on top of a 15 foot pole. A person who is 6 feet tall walks away from the pole at a rate of 4 feet per second. At what speed is the length of the person’s shadow growing? 1. speed = 23 9 ft/sec mcguire (am89323) – 2.6 Related Rates – toshner – (AB-4) 4 2. speed = 3 ft/sec 3. speed = 25 9 ft/sec 4. speed = 8 3 ft/sec 5. speed = 26 9 ft/sec

Answer 1

(4). 8/3 ft/sec.

Speed of person's shadow growing = 2.66 ft/sec.

Step-by-step explanation:

In the question,

The height of the pole is = 15 foot

Height of the person = 6 foot

Rate of walking away from the pole, v = dy/dt = 4 ft/sec.

Now,

Let us say the length of shadow is = x

and,

Distance of person from the pole is = y

So,

In the triangle EDC and EAB, from the similar triangle properties, we can say,

`(EC)/(EB)=(CD)/(AB)\\(x)/(x+y)=(6)/(15)\\5x=2x+2y\\3x=2y`

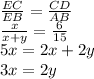
Now,

On differentiating the equation w.r.t, time, t, we get,

`3x=2y\\3(dx)/(dt)=2(dy)/(dt)\\Now,\\(dy)/(dt)=4\\So,\\(dx)/(dt)=(2)/(3)(4)=(8)/(3)\\(dx)/(dt)=2.66\,ft/sec.`

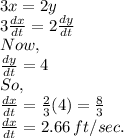
Therefore, the Speed at which the person's shadow is growing is 2.66 ft/sec.

Hence, the correct option is (4).