Question

If an investment of $45,000 is earning an interest rate of 8.50% compounded annually, it will take for this investment to grow to a value of $57,478.01—assuming that no additional deposits or withdrawals are made during this time? Which of the following statements is true, assuming that no additional deposits or withdrawals are made? If you invest $1 today at 15% annual compound interest for 82.3753 years, you’ll end up with $100,000. If you invest $5 today at 15% annual compound interest for 82.3753 years, you’ll end up with $100,000.

Answer 1

(A) 3 years.

(B) True

(C) False

Step-by-step explanation:

We will use compound interest formula to solve our given problems.

`A=P(1+(r)/(n))^(nt)`, where,

A = Final amount after t years,

P = Principal amount,

r = Annual interest rate in decimal form,

n = Number of times period is compounded per year,

t = Time in years.

(A)

`8.50\%=(8.50)/(100)=0.085`

`\$57,478.01=\$45,000(1+(0.085)/(1))^(1*t)`

`\$57,478.01=\$45,000(1+0.085)^(t)`

`\$57,478.01=\$45,000(1.085)^(t)`

`(\$57,478.01)/(\$45,000)=(\$45,000(1.085)^(t))/(\$45,000)`

`1.2772891111=1.085^(t)`

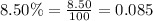
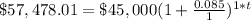
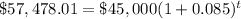
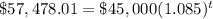
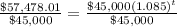
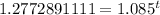
Take natural log of both sides:

`\text{ln}(1.2772891111)=\text{ln}(1.085^(t))`

`\text{ln}(1.2772891111)=t\cdot\text{ln}(1.085)`

`\frac{\text{ln}(1.2772891111)}{\text{ln}(1.085)}=\frac{t\cdot\text{ln}(1.085)}{\text{ln}(1.085)}`

`(0.2447399500948)/(0.0815799869924229)=t`

`2.99999=t`

`t=2.99999`

`t\approx 3`

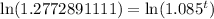
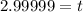
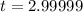
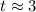
Therefore, it will take approximately 3 years for the investment to grow to a value of $57,478.01.

(B)

`15\%=(15)/(100)=0.15`

`A=\$1(1+(0.15)/(1))^(1*82.3753)`

`A=\$1(1+0.15)^(82.3753)`

`A=\$1(1.15)^(82.3753)`

`A=\$1*100,000.6466787091401`

`A\approx \$100,000`

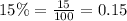
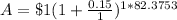
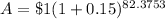
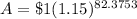
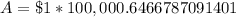
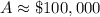
Since the final amount is approximately $100,000, therefore, the given statement is true.

(C)

`15\%=(15)/(100)=0.15`

`A=\$5(1+(0.15)/(1))^(1*82.3753)`

`A=\$5(1+0.15)^(82.3753)`

`A=\$5(1.15)^(82.3753)`

`A=\$5*100,000.6466787091401`

`A\approx \$500,003`

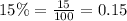
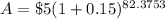
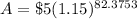
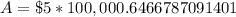
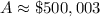
Since the final amount is approximately $500,000, therefore, the given statement is false.