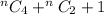Question

N points lie on a circle. You draw lines connecting all the points to each other. These lines divide up the circle into a number of regions. How many regions is this? Assume that the points are scattered in such a way as to give the maximum number of regions for that N.

Answer 1

`Number\,of\,regions=^nC_(4)+^nC_(2)+1`

Explanation:

In the question,

There are 'n' points on the circle.

For making the maximum number of regions we can do that by selecting 2 points from the given number of points.

i.e.
`^nC_(2)`

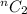
And,

By selecting 4 points from the given number of points we get the extra regions formed on the intersection of the chords with each other.

i.e.
`^nC_(4)`

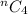
And, 1 more region.

So, we can write it as,

`Number\,of\,regions=^nC_(4)+^nC_(2)+1`

Therefore, the number of regions formed from the 'N' points are,

`^nC_(4)+^nC_(2)+1`