Question

(a) If the maximum acceleration that is tolerable for passengers in a subway train is 1.44 m/s2 and subway stations are located 846 m apart, what is the maximum speed a subway train can attain between stations? (b) What is the travel time between stations? (c) If a subway train stops for 22.0 s at each station, what is the maximum average speed of the train, from one start-up to the next?

Answer 1

(a): 34.90 m/s.

(b): 48.48 s.

(c): 12.00 m/s.

Step-by-step explanation:

We have,

• The maximum acceleration that the train could attain between the two stations,
  `\rm a = 1.44\ m/s^2.`
• Distance between the subways, s = 846 m.

Assuming the initial and the final velocity of the train between the two stations be u and v respectively.

The train started from rest, therefore, u = 0 m/s.

(a):

Note: To find the maximum speed attained by the train, we have to consider the distance which is half to the distance between the stations, otherwise the train would attain the maximum speed at the second stations which make it impossible to stop the train with that acceleration.

If the train attain maximum velocity at half of the that distance then train will start decelerating so that it comes to rest at the second station.

Let,
`\rm x=\frac s2=(846)/(2)=423\ m.`

Using the following equation of the Kinematics,

`\rm v^2-u^2=2as\\v^2-o^2=2* 1.44* 423=1218.24\\v=34.90\ m/s.`

It is the maximum speed the train can attain.

(b):

The travel time t of the train to cover half the distance between stations is given by

`\rm v=u+at\\t=(v-u)/(a)=(34.90-0)/(1.44)=24.24\ s.`

It is the time taken by the train to cover half of the distance between the stations.

The total time taken by the train to cover the whole distance between the stations =
`2* 24.24 = 48.48\ \rm s.`

(c):

The average speed of the train is given by the total distance traveled by the train divided by the total time taken by the train.

The total time taken by the train from one start-up to next is the sum of the time taken by the train to move from one station to another and the time for which the train stops.

`\rm t_(total)= t+22 = 48.48+22 = 70.48\ s.`

Therefore, average velocity =
`(846)/(70.48)=12.00\ \rm m/s.`

Answer 2

(a) 34.90 m/s

(b) 48.48 s

(c) 12 m/s

Step-by-step explanation:

Given:

• a = Maximum acceleration tolerable by the passengers =
  `1.44\ m/s^2`
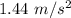
• x = distance between two stations = 846 m
• T = time of stoppage at each station = 22.0 s
• u = initial speed of the train = 0 m/s

For the maximum velocity attained by the subway train and also to stop at the destination (next station), the train must have to accelerate with a constant acceleration of
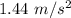`1.44\ m/s^2` for half the journey to reach its maximum attainable velocity and then decelerate with
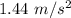`1.44\ m/s^2` to stop at the next stage for the remaining half journey.

So, let us assume

• v = maximum speed of the train
• s = distance traveled to attain that maximum speed =
  `(x)/(2)=(846\ m)/(2) = 423\ m`
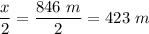
• t = time interval in which the maximum speed is attained

Part (a):

Using the equation for constant acceleration motion, we have

`v^2=u^2+2as\\\Rightarrow v^2=(0)^2+2(1.44)(423)\\\Rightarrow v^2 = 1218.24\\`

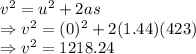
taking square root on both sides

`\Rightarrow v=\pm √(1218.24)\\\Rightarrow v=\pm 34.90\\`

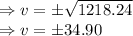
Since, speed of the train not be negative for the journey.

`\therefore v = 34.90`

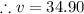
Hence, the train attains a maximum speed of 34.90 m/s between the two stations.

Part (b):

Let us first find out the time taken by the train to reach its maximum speed.

`v = u+at\\\Rightarrow t = (v-u)/(a)\\\Rightarrow t = (34.90-0)/(1.44)\\\Rightarrow t = 24.24`

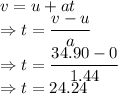
This means it takes 24.24 s to reach its maximum speed in the half journey.

Since the magnitude of acceleration and deceleration in both the half journey is the same. So, time taken by the train in both the journey will be the same.

So, the total travel time between the two stations is double the time travel in half the journey i.e., 48.48 s.

Hence, the travel time between stations is 48.48 s.

Part (c):

The train starts up from the originating one station and reaches the second station in the travel time (48.48 s) to wait for another 22.0 s at the destination. So, Total time = 48.48 s + 22.0 s = 70.48 s.

Now

`\textrm{The maximum average speed of the train}=\frac{\textrm{Total distance}}{\textrm{Total time}}\\\Rightarrow V_(avg) = (846\ m)/(70.48\ s)\\\Rightarrow V_(avg) = 12.0\ m/s`

Hence, the maximum average speed of the train from one start-up to the next is 12.0 m/s.