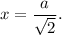The electric field intensity as a function of the distance
 from the center of the ring is given by:
from the center of the ring is given by:
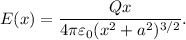
Taking the derivative of
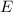 with respect to
with respect to
 , we get:
, we get:
![\frac{\textrm{d}E}{\textrm{d}x} = (Q)/(4\pi\varepsilon_0)\frac{\textrm{d}}{\textrm{d}x}\left[(x)/((x^2 + a^2)^(3/2))\right].](https://img.qammunity.org/2020/formulas/physics/college/6l6ura116tctv4ace9gs3gcb2fyv935f5i.png)
We will now use the quotient rule:
![\frac{\textrm{d}}{\textrm{d}x}\left[(x)/((x^2 + a^2)^(3/2))\right] = \frac{\frac{\textrm{d}x}{\textrm{d}x}(x^2+a^2)^(3/2) - x\frac{\textrm{d}}{\textrm{d}x}\left[(x^2+a^2)^(3/2)\right]}{\left[(x^2+a^2)^(3/2)\right]^2}.](https://img.qammunity.org/2020/formulas/physics/college/yc5q8mpgqelui2tg7izalrorirhjl5ypw3.png)
We now use the chain and power rules to get:
![\frac{\textrm{d}}{\textrm{d}x}\left[(x^2+a^2)^(3/2)\right] = (3)/(2)(x^2+a^2)^(1/2)\frac{\textrm{d}}{\textrm{d}x}(x^2 + a^2) = (3)/(2)√(x^2 + a^2)2x = 3x√(x^2 + a^2).](https://img.qammunity.org/2020/formulas/physics/college/sam3z40tj5t85zuwxnxm7ne1h2dntwu4qn.png)
And also:
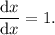
The derivative is then:
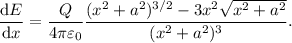
Since the denominator is never zero and
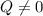 , we can write:
, we can write:
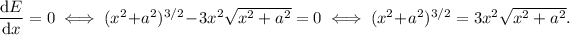
Dividing both sides of the equation by
 , we get:
, we get:

We now need to check whether this is a maximum, a minimum or a saddle point. We can use the second derivative test, but there's an easier way. Since
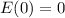 ,
,
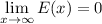 and
and
 for
for
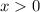 ,
,
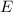 necessarily has a maximum, since
necessarily has a maximum, since
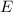 is continuous.
is continuous.
Since we want positive values of
 , the solution is:
, the solution is: