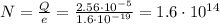Question

Two balloons (m = 0.021 kg) are separated by a distance of d = 16 m. They are released from rest and observed to have an instantaneous acceleration of a = 1.1 m/s2 toward each other at the moment they are released. Assume the two balloons are point charges and have equal but opposite charges

(a) Calculate the magnitude of the charge on each balloon in C.
(b) How many electrons are responsible for the charge, N?

Answer 1

(a)
`2.56\cdot 10^(-5) C`

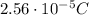
According to Newton's second law, the force experienced by each balloon is given by:

F = ma

where

m = 0.021 kg is the mass

a = 1.1 m/s^2 is the acceleration

Substituting, we found:

`F=(0.021)(1.1)=0.0231 N`

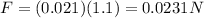
The electrostatic force between the two balloons can be also written as

`F=k(Q^2)/(r^2)`

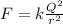
where

k is the Coulomb's constant

Q is the charge on each balloon

r = 16 m is their separation

Since we know the value of F, we can find Q, the magnitude of the charge on each balloon:

`Q=\sqrt{(Fr^2)/(k)}=\sqrt{((0.0231)(16)^2)/(9\cdot 10^9)}=2.56\cdot 10^(-5) C`

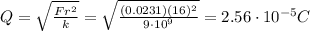
(b)
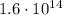`1.6\cdot 10^(14)` electrons

The magnitude of the charge of one electron is

`e=1.6\cdot 10^(-19)C`

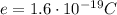
While the magnitude of the charge on one balloon is

`Q=2.56\cdot 10^(-5) C`

This charge can be written as

`Q=Ne`

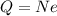
where N is the number of electrons that are responsible for this charge. Solving for N, we find:

`N=(Q)/(e)=(2.56\cdot 10^(-5))/(1.6\cdot 10^(-19))=1.6\cdot 10^(14)`