Explanation:
We have given,

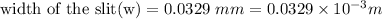
distance of the slit from the screen(d) = 3.2m
distance from the central maximum(m) = 1.3cm = 0.013m
Now,
(a). The expression for the angle of the inclination of the wave is (
 )
)
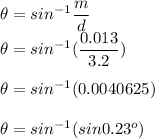
Thus the angle of inclination of the wave is
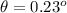
(b). The expression for the angle


after solving the equation we get,
Thus the angle
 = 0.61 radian
= 0.61 radian
(c). the expression for the ratio of the intensity is
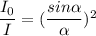
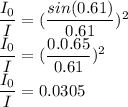
Thus the ratio of the intensity is 0.0305