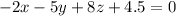Question

Find an equation of the plane consisting of all points that are equidistant from (-3, 5, -4) and (-5, 0, 4), and having -2 as the coefficient of x

Answer 1

`-2x-5y+8z+4.5=0`

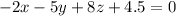
Explanation:

Let an equation of the plane

`ax+by+cz+d=0`

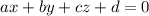
We have to find the equation of the plane consisting of all points that are equidistant from (-3,5,-4) and (-5,0,4).

The coefficient of x=-2

Distance formula=
`√((x_2-x_1)^2+(y_2-y_1)^2+(z_2-z_1)^2)`

Let
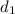`d_1` be the distance of point (x,y,z)lie on the plane and point (-3,5,-4).

`d_1=√((x+3)^2+(y-5)^2+(z+4)^2)`

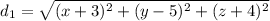
Let
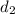`d_2` be the distance between the point (x,y,z) lie on the plane and the point (-5,0,4).

`d_2=√((x+5)^2+y^2+(z-4)^2)`

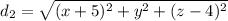
According to question

`d_1=d_2`

`√((x+3)^2+(y-5)^2+(z+4)^2)=√((x+5)^2+y^2+(z-4)^2)`

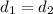
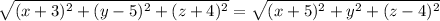
Squaring on both sides

`(x+3)^2+(y-5)^2+(z+4)^2=(x+5)^2+y^2+(z-4)^2`

`x^2+6x+9+y^2-10y+25+z^2+8z+16=x^2+10x+25+y^2+z^2-8z+16`

`x^2+6x+50+y^2-10y+z^2+8z-x^2-10x-z^2-y^2+8z-41=0`

`-4x-10y+16z+9=0`

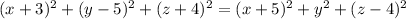
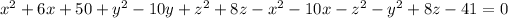
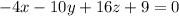
Divided the equation by 2

`-2x-5y+8z+4.5=0`

Answer 2

-2x-5y+8z+4.5=0

Explanation:

Let (x,y,z) be the coordinates of the point lying on the needed plane. This point is equidistant from the points (-3, 5, -4) and (-5, 0, 4), so

`d_1=√((x-(-3))^2+(y-5)^2+(z-(-4))^2)=√((x+3)^2+(y-5)^2+(z+4)^2)\\ \\d_2=√((x-(-5))^2+(y-0)^2+(z-4)^2)=√((x+5)^2+y^2+(z-4)^2)\\ \\d_1=d_2\Rightarrow √((x+3)^2+(y-5)^2+(z+4)^2)=√((x+5)^2+y^2+(z-4)^2)\\ \\(x+3)^2+(y-5)^2+(z+4)^2=(x+5)^2+y^2+(z-4)^2\\ \\x^2+6x+9+y^2-10y+25+z^2+8z+16=x^2+10x+25+y^2+z^2-8z+16\\ \\-4x-10y+16z+9=0\\ \\-2x-5y+8z+4.5=0`