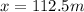Answer:
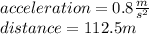
Step-by-step explanation:
We can find these answers following the equations of motion.
- To find the acceleration, we use the equation:
 Δ
Δ
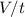
Where Δ
 is the difference between the final speed and the initial speed. And
is the difference between the final speed and the initial speed. And
 is the time spent
is the time spent
We replace the terms:
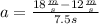
We solve the difference:
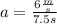
We divide the terms, so we can have the answer:
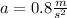
2. To find the distance traveled by the truck, we use the equation:
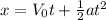
Where
 is the distance traveled,
is the distance traveled,
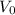 is the initial speed,
is the initial speed,
 is the acceleration and
is the acceleration and
 is the time.
is the time.
We replace the terms:
![x=(12(m)/(s)*7.5s)+(1)/(2)[0.8(m)/(s^(2) )*(7.5)^(2) ]](https://img.qammunity.org/2020/formulas/physics/college/rire6fe7eyd1epr3n1ye9uj7twkgfdfcnt.png)
We multiply and solve the exponential:
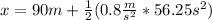
Then, we multiply the terms left:
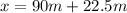
And add, so we can have the answer: