Question

A diverging lens has a focal length of 23.9 cm. An object 2.1 cm in height is placed 100 cm in front of the lens. Locate the position of the image. Answer in units of cm. 007 (part 2 of 3) 10.0 points What is the magnification? 008 (part 3 of 3) 10.0 points Find the height of the image. Answer in units of cm.

Answer 1

Image is virtual and formed on the same side as the object, 19.29 cm from the lens.

The height of the image is 0.40509 cm

Image is upright as the magnification is positive and smaller than the object.

Step-by-step explanation:

u = Object distance = 100 cm

v = Image distance

f = Focal length = -23.9 cm (concave lens)

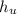`h_u`= Object height = 2.1 cm

Lens Equation

`(1)/(f)=(1)/(u)+(1)/(v)\\\Rightarrow (1)/(f)-(1)/(u)=(1)/(v)\\\Rightarrow (1)/(v)=(1)/(-23.9)-(1)/(100)\\\Rightarrow (1)/(v)=(-1239)/(23900) \\\Rightarrow v=(-23900)/(1239)=-19.29\ cm`

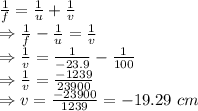
Image is virtual and formed on the same side as the object, 19.29 cm from the lens.

Magnification

`m=-(v)/(u)\\\Rightarrow m=-(-19.29)/(100)\\\Rightarrow m=0.1929`

`m=(h_v)/(h_u)\\\Rightarrow 0.1929=(h_v)/(2.1)\\\Rightarrow h_v=0.1929* 2.1=0.40509\ cm`

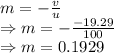
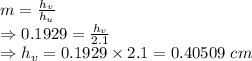
The height of the image is 0.40509 cm

Image is upright as the magnification is positive and smaller than the object.