Answer:
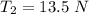
Step-by-step explanation:
Given that,
Speed of transverse wave, v₁ = 20 m/s
Tension in the string, T₁ = 6 N
Let T₂ is the tension required for a wave speed of 30 m/s on the same string. The speed of a transverse wave in a string is given by :
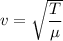 ........(1)
........(1)
T is the tension in the string
 is mass per unit length
is mass per unit length
It is clear from equation (1) that :
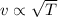
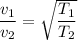
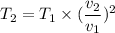
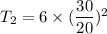
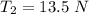
So, the tension of 13.5 N is required for a wave speed of 30 m/s. Hence, this is the required solution.