Question

Find an equation of a line passing through the point (8,9) and parallel to the line joining the points (2,7) and (1,5).

Answer 1

y=2x-8

Explanation:

In order to solve this you first have to calculate the slope of the parallel line, since that would be equal to the slope of our line:

`Slope=(y2-y1)/(x2-x1)`

Now we insert the values into the formula:

`Slope=(y2-y1)/(x2-x1)\\Slope=(5-7)/(1-2)\\Slope= (-2)/(-1)\\ Slope:2`

And remember that the formula for general line is:

`Y-y1= M(x-x1)\\y-9=2(x-8=\\y=2x-16+9\\y=2x-7`

So the equation for the line passing through point 8,9 and parallel to the line joining 2,7 and 1,5 would be y=2x-7

Answer 2

2x - y - 7 = 0

Explanation:

Since the slope of parallel line are same.

So, we can easily use formula,

y - y₁ = m ( x ₋ x₁)

where, (x₁, y₁) = (8, 9)

and m is a slope of line passing through (x₁, y₁).

and since the slope of parallel lines are same, so here we use slope of parallel line for calculation.

and, Slope = m =
`(y_(b)-y_(a))/(x_(b)-x_(a))`

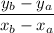
here, (xₐ, yₐ) = (2, 7)

and,
`(y_(a),y_(b)) = (1, 5 )`

⇒ m =
`(5-7)/(1-2)`

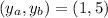
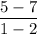
⇒ m = 2

Putting all values above formula. We get,

y - 9 = 2 ( x ₋ 8)

⇒ y - 9 = 2x - 16

⇒ 2x - y - 7 = 0

which is required equation.