Question

There are two parallel conductive plates separated by a distance d and zero potential. Calculate the potential and electric field that occurs if a q charge is placed between the plates at a distance d/2.

Answer 1

The total electric potential at mid way due to 'q' is
`(q)/(4\pi\epsilon_(o)d)`

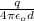
The net Electric field at midway due to 'q' is 0.

Solution:

According to the question, the separation between two parallel plates, plate A and plate B (say) = d

The electric potential at a distance d due to 'Q' is:

`V = (1)/(4\pi\epsilon_(o)).(Q)/(d)`

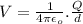
Now, for the Electric potential for the two plates A and B at midway between the plates due to 'q':

For plate A,

`V_(A) = (1)/(4\pi\epsilon_(o)).(q)/((d)/(2))`

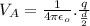
Similar is the case with plate B:

`V_(B) = (1)/(4\pi\epsilon_(o)).(q)/((d)/(2))`

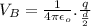
Since the electric potential is a scalar quantity, the net or total potential is given as the sum of the potential for the two plates:

`V_(total) = V_(A) + V_(B) = (1)/(4\pi\epsilon_(o)).q((1)/((d)/(2)) + (1)/((d)/(2))`

`V_(total) = (q)/(4\pi\epsilon_(o)d)`

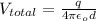
Now,

The Electric field due to charge Q at a distance is given by:

`\vec{E} = (1)/(4\pi\epsilon_(o)).(Q)/(d^(2))`

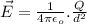
Now, if the charge q is mid way between the field, then distance is
`(d)/(2)`.

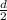Electric Field at plate A,
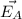`\vec{E_(A)}` at midway due to charge q:

`\vec{E_(A)} = (1)/(4\pi\epsilon_(o)).(q)/(((d)/(2))^(2))`

Similarly, for plate B:

`\vec{E_(B)} = (1)/(4\pi\epsilon_(o)).(q)/(((d)/(2))^(2))`

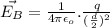
Both the fields for plate A and B are due to charge 'q' and as such will be equal in magnitude with direction of fields opposite to each other and hence cancels out making net Electric field zero.