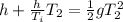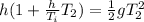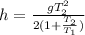Question

An elevator ascends from the ground with uniform speed. A time Tį later, a boy drops a marble through a hole in the floor. A time T2 after that (i.e. Ti +T2 after start) the marble hits the ground. Find an expression for the height of the elevator at 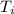time Ti. (Local gravity is g.) What checks can you make?

Answer 1

The height of the elevator at
`T_(i)` is
`(gT_(2)^(2))/(2(1 + (T_(2))/(T_(i))))`

Solution:

As per the question:

Let us assume:

The velocity with which the elevator ascends be u'

The height attained by the elevator at time,
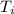`T_(i)` be h

Thus

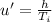`u' = (h)/(T_(i))` (1)_

Now, with the help of eqn (2) of motion, we can write:

`h = - u'T_(2) + (1)/(2)gT_(2)^(2)`

Using eqn (1):

`h = - (h)/(T_(i))T_(2) + (1)/(2)gT_(2)^(2)`

`h + (h)/(T_(i))T_(2) = (1)/(2)gT_(2)^(2)`

`h(1 + (h)/(T_(i))T_(2)) = (1)/(2)gT_(2)^(2)`

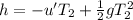
`h = (gT_(2)^(2))/(2(1 + (T_(2))/(T_(1))))`