Question

If 2^n + 1 is an odd prime for some integer n, prove that n is a power of 2. (H

Answer 1

Explanation:

We will prove by contradiction. Assume that
`2^n + 1` is an odd prime but n is not a power of 2. Then, there exists an odd prime number p such that
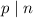`p\mid n`. Then, for some integer
`k\geq 1`,

`n=p* k.`

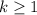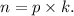
Therefore

1. 
   `2^n + 1=2^(p* k) + 1=(2^(k))^p + 1^p.`

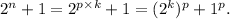
Here we will use the formula for the sum of odd powers, which states that, for
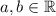`a,b\in \mathbb{R}` and an odd positive number
`n`,

`a^n+b^n=(a+b)(a^(n-1)-a^(n-2)b+a^(n-3)b^2-...+b^(n-1))`

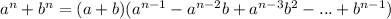
Applying this formula in 1) we obtain that

`2^n + 1=2^(p* k) + 1=(2^(k))^p + 1^p=(2^k+1)(2^(k(p-1))-2^(k(p-2))+...-2^(k)+1)`.

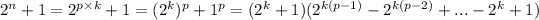Then, as
`2^k+1>1` we have that
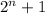`2^n+1` is not a prime number, which is a contradiction.

In conclusion, if
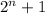`2^n+1` is an odd prime, then n must be a power of 2.