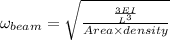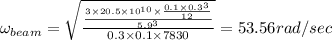Answer:
The natural angular frequency of the rod is 53.56 rad/sec
Step-by-step explanation:
Since the beam is free at one end and fixed at the other hence the beam is a cantilevered beam as shown in the attached figure
We know that when a unit force is placed at the end of a cantilever the displacement of the free end is given by
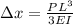
Hence we can write

Comparing with the standard spring equation
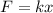 we find the cantilever analogous to spring with
we find the cantilever analogous to spring with
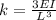
Now the angular frequency of a spring is given by
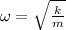
where
'm' is the mass of the load
Thus applying values we get