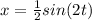Answer:
a) x=(t^2)/2+cos(t), b) x=2+3e^(-2t), c) x=(1/2)sin(2t)
Explanation:
Let's solve by separating variables:
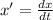
a) x’=t–sin(t), x(0)=1
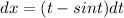
Apply integral both sides:
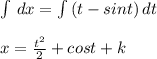
where k is a constant due to integration. With x(0)=1, substitute:
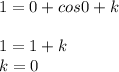
Finally:
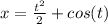
b) x’+2x=4; x(0)=5
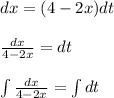
Completing the integral:
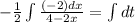
Solving the operator:
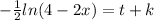
Using algebra, it becomes explicit:
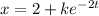
With x(0)=5, substitute:
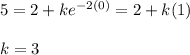
Finally:
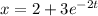
c) x’’+4x=0; x(0)=0; x’(0)=1
Let
 be the solution for the equation, then:
be the solution for the equation, then:
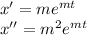
Substituting these equations in c)

This becomes the solution m=α±βi where α=0 and β=2
![x=e^(\alpha t)[Asin\beta t+Bcos\beta t]\\\\x=e^(0)[Asin((2)t)+Bcos((2)t)]\\\\x=Asin((2)t)+Bcos((2)t)](https://img.qammunity.org/2020/formulas/mathematics/college/pszaf9ecnj588s9irfc2kdkktpraf8n969.png)
Where A and B are constants. With x(0)=0; x’(0)=1:
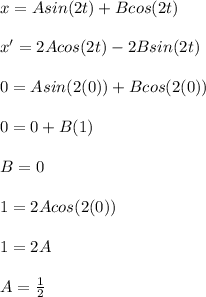
Finally: