Answer:
The wavelength of the wave is 20 m.
Step-by-step explanation:
Given that,
Amplitude = 10 cm
Radial frequency
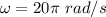
Bulk modulus = 40 MPa
Density = 1000 kg/m³
We need to calculate the velocity of the wave in the medium
Using formula of velocity
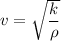
Put the value into the formula
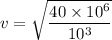
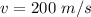
We need to calculate the wavelength
Using formula of wavelength
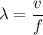
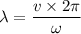
Put the value into the formula
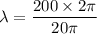
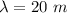
Hence, The wavelength of the wave is 20 m.