Step-by-step explanation:
The chemical reaction is as follows.
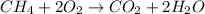
It is given that 2094
 . And, it is known that 1
. And, it is known that 1
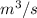 = 127133
= 127133

Hence, convert 2094
 into
into
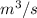 as follows.
as follows.
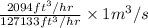
=
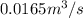
As ideal gas equation is PV = nRT. So, calculate the number of moles as follows.
n =

=
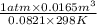
= 0.673 mol/sec
According to the stoichiometry of the given reaction, 1 mol of methane reacts with 2 mol of oxygen.
So, 1 mol
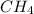 = 2 mol
= 2 mol
![O_(2)[\tex]</p><p>Hence, [tex]O_(2)[\tex] required theoretically = [tex]2 * 0.673 mol/s](https://img.qammunity.org/2020/formulas/chemistry/college/lo1rcdcwxphzh9nzy3xfhh2wacaflm7jip.png) = 1.346 mol/s
= 1.346 mol/s
Hence, air required theoretically =
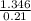 = 6.4095 mol/s.
= 6.4095 mol/s.
Since, 6% of excess air is being supplied. Therefore, total air supplied will be calculated as follows.
Total air supplied =
![6.4095 mol/s [1 + (6)/(100)]](https://img.qammunity.org/2020/formulas/chemistry/college/ftd6jis9rjodekqegl0stfttbod9jvu0ds.png)
= 6.794 mol/s
Now, calculate the volume using ideal gas law equation as follows.
PV = nRT
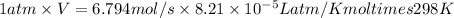
V = 0.166229
Converting calculated volume into
 as follows.
as follows.
1
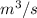 = 127133
= 127133

So, 0.166229
 =
=
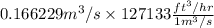
= 21133.191

Thus, we can conclude that 21133.191
 of air are drawn from outside per hour by the fan that supplies the air.
of air are drawn from outside per hour by the fan that supplies the air.