Answer:
(a). The average speed is 97.23 km/hr.
(b). The average velocity is 81.70 km/min.
Step-by-step explanation:
Given that,
Distance in east = 14.5 km
Distance in south = 66.5 km
Time = 50 min = 0.833 hr
(a). We need to calculate the average speed
Using formula of average speed
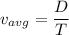
Where, D = total distance
T = total time
Put the value into the formula
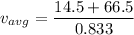
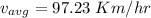
(b). We need to calculate the displacement
Using Pythagorean theorem
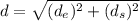
Put the value into the formula
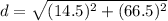
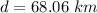
(b). We need to calculate the average velocity
Using formula of average velocity

Where, d = displacement
t = time
Put the value into the formula
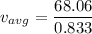
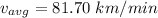
Here, We can not necessary to find the unit vectors for displacement because we need to the displacement for find the average velocity.
Hence, (a). The average speed is 97.23 km/hr.
(b). The average velocity is 81.70 km/hr.