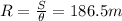Answer:
R = 3170.36m or R = 186.5m
Step-by-step explanation:
For this problem, we have either trajectory (a), assuming that the car was going south-east, or trajectory (b), assuming the car was going north-east.
In both cases, we know that S = 830m = θ * R. Finding θ, will lead us to the value of R.
For option a:
θ = 15° = 0.2618 rad
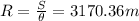
For option b:
θ = 270° - 15° = 4.45 rad