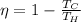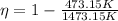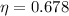1 Answer

Daphna · Answer 1 · 2020-03-27T06:35:45+0000

Answer:

efficiency = 0.678

Step-by-step explanation:

First we have to change temperatures from °C to K (always in thermodynamics absolute temperature is used).

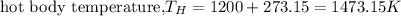
$\text{hot body temperature,}T_H = 1200 \circC + 273.15 = 1473.15 K$

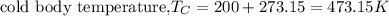
$\text{cold body temperature,} T_C = 200 \circC + 273.15 = 473.15 K$

Efficiency
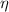$\eta$ of a carnot engine can be calculated only with hot body and cold body temperature by

$\eta = 1 - (T_C)/(T_H)$

$\eta = 1 - (473.15 K)/(1473.15 K)$

$\eta = 0.678$

Please log in or register to add a comment.