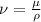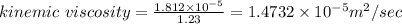Question

Calculate the viscosity(dynamic) and kinematic viscosity of airwhen

the temperature is 288.15K and the density is 1.23kg/m3.

Answer 1

(a) dynamic viscosity =
`1.812* 10^(-5)Pa-sec`

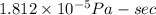
(b) kinematic viscosity =
`1.4732* 10^(-5)m^2/sec`

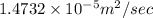
Step-by-step explanation:

We have given temperature T = 288.15 K

Density
`d=1.23kg/m^3`

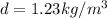
According to Sutherland's Formula dynamic viscosity is given by

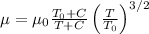`{\mu} = {\mu}_0 \frac {T_0+C} {T + C} \left (\frac {T} {T_0} \right )^(3/2)`, here

μ = dynamic viscosity in (Pa·s) at input temperature T,

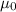`\mu _0`= reference viscosity in(Pa·s) at reference temperature T0,

T = input temperature in kelvin,

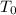`T_0` = reference temperature in kelvin,

C = Sutherland's constant for the gaseous material in question here C =120

`\mu _0=4\pi * 10^(-7)`

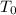`T_0` = 291.15

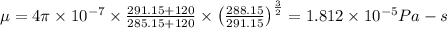`\mu =4\pi * 10^(-7)* (291.15+120)/(285.15+120)* \left ( (288.15)/(291.15) \right )^{(3)/(2)}=1.812* 10^(-5)Pa-s`when T = 288.15 K

For kinematic viscosity :

`\\u = \frac {\mu} {\rho}`

`kinemic\ viscosity=(1.812* 10^(-5))/(1.23)=1.4732* 10^(-5)m^2/sec`