Answer:
Line A is represented by the function f(x) = 2x + 1.
Explanation:
This can be solved by trial and error. What it means? It means that we are going to replace the ordered pairs in the function, and the equality must be satisfied.
Line A
(-2,-3)
When x = -2, f(x) = -3. Does it happen in the function?
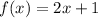
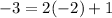
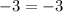
The first equality is OK. Let's see the second
(2,5)
When x = 2, y = 5.
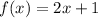
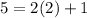
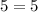
Also OK.
So line A is represented by the function f(x) = 2x + 1.
Now let's see why the other lines are not represented by this function.
Line B
(-2,-4)
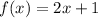
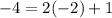
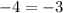
False
Line C
(-2,-5)
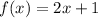
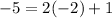
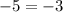
False
Line D
(0,-4)
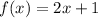
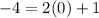
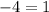
False