Question

PLEASE HELP!!!

Aleko’s Pizza has delivered a beautiful 16 inch diameter pie to Lee dorm room. The pie is slice into 8 equal sizes pieces, but Lee is such a non-conformist he cuts off an edge as pictured. John then takes on e of the remaining triangular slices. Who has more pizza and by how much?

Answer 1

Without exact dimensions of Lee's cut, we can't calculate the precise difference in area after he cuts off an edge, but John likely has more pizza since his slice is unmodified.

Step-by-step explanation:

The student's question involves comparing areas of pizza slices after one has been modified by cutting off an edge. To answer who has more pizza and by how much, we need to calculate the area of the pizza slices. The original pizza is 16 inches in diameter, and when divided into 8 equal slices, each slice is a sector of a circle with a central angle of 45 degrees. If Lee cuts off an edge and John takes an unmodified triangular slice, John would likely have more pizza because Lee's slice has been reduced in size. However, without knowing the exact dimensions of the removed edge, we can't calculate the precise difference in area.

]

Answer 2

Lee has more pizza

Lee has 2.24 in^2 more than John

Step-by-step explanation:

step 1

Find the area of each slice of pizza

`A=(1)/(8)\pi r^(2)`

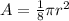
we have

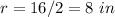`r=16/2=8\ in` ----> the radius is half the diameter

substitute

`A=(1)/(8)\pi 8^(2)`

`A=8\pi\ in^(2)`

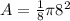
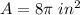
step 2

Find the area of John's part (area of shaded triangle)

The measure of the central angle of each slice of pizza is equal to

`360\°/8=45\°`

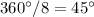
so

the height of triangle is equal to the base

Let

x ---->the base of the shaded triangle

`cos(45\°)=(x)/(r)`

`cos(45\°)=(x)/(8)`

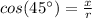
Remember that

`cos(45\°)=(√(2))/(2)`

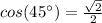
substitute

`(√(2))/(2)=(x)/(8)`

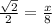
solve for x

`x=4√(2)\ in`

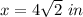
Find the area of shaded triangle

`A=(1/2)(4√(2))(4√(2))=16\ in^2`

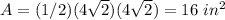
step 3

Find the area of Lee's part

The area of Lee's part is equal to the area of two slices of pizza minus the area of two triangles

so

`2(8\pi)-2(16)=(16\pi-32)\ in^2`

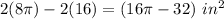
assume

`\pi =3.14`

`(16(3.14)-32)=18.24\ in^2`

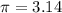
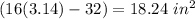
so

Lee's part is greater than John part's

Find the difference

`18.24-16=2.24\ in^2`

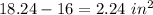
therefore

Lee has more pizza

Lee has 2.24 in^2 more than John