Answer: There are 18 players who played one or more of the three sports.
Explanation:
Since we have given that
Number of students played basketball = 7
Number of students played volleyball = 9
Number of students played soccer = 10
Number of students played basketball and volleyball = 1
Number of students played volleyball and soccer = 2
Number of students played volleyball, basketball and soccer = 2
Number of students who played basketball only is given by
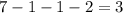
Number of students who played volleyball only is given by

Number of students who played soccer only is given by
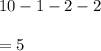
So, Number of students one or more of the three sports is given by
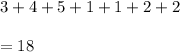
Hence, there are 18 players who played one or more of the three sports.