Answer:
The squirrel's initial velocity is 5 m/s.
Step-by-step explanation:
It is given that,
Distance covered by the squirrel, d = 5 m
Time taken, t = 0.5 s
Final speed of the squirrel, v = 15 m/s
To find,
The squirrel's initial velocity.
Solution,
Let a is the acceleration of the squirrel. Using the first equation of motion to find it :
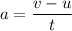
 ..............(1)
..............(1)
Let u is the initial speed of the squirrel. Using again third equation of kinematics to find it :
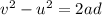
Use equation (1) to put value of a
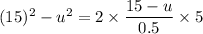

On solving the above quadratic equation, we get the value of u as :
u = 5 m/s
Therefore, the squirrel's initial velocity before getting started is 5 m/s.