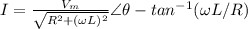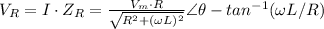Answer:
The equations for magnitude and phase of current and voltages on resistor and inductor are:
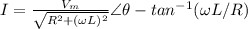
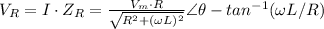

Step-by-step explanation:
The first step is to find the impedances of the resistance (R) and the inductor (L).
The impedance of the resistor is:
- Rectangular form:

- Polar form:
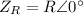
The impedance of the inductor is:
- Rectangular form:
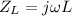
- Polar form:
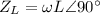
Where
 is the angular frequency of the source, and the angle is
is the angular frequency of the source, and the angle is
 because a pure imaginary number is on the imaginary axis (y-axis).
because a pure imaginary number is on the imaginary axis (y-axis).
The next step is to find the current expression. It is the same for the resistor and inductor because they are in series. The total impedance equals the sum of each one.
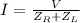
It is said that
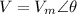 , so, the current would be:
, so, the current would be:
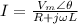
The numerator must be converted to polar form by calculating the magnitude and the angle:
- The magnitude is

- The angle is
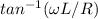
The current expression would be as follows:
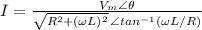
When dividing, the angles are subtracted from each other.
The final current expression is:
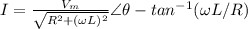
The last step is calculating the voltage on the resistor
 and the voltage on the inductor
and the voltage on the inductor
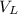 . In this step the polar form of the impedances could be used. Remember that
. In this step the polar form of the impedances could be used. Remember that
 .
.
(Also remember that when multiplying, the angles are added from each other)
Voltage on the resistor

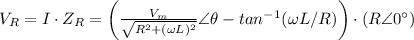
The final resistor voltage expression is:
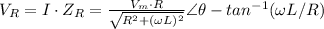
Voltage on the inductor
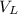
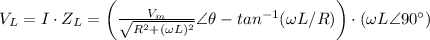
The final inductor voltage expression is:

Summary: the final equations for magnitude and phase of current and voltages on resistor and inductor are: