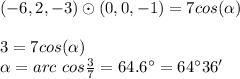Answer:
The tower deviates
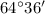 from the vertical.
from the vertical.
Explanation:
Having 3 point of our new plane we can construct vectors on the plane by substacting 2 of them:
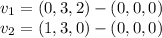
These vectors are on the plane, so a cross product between them will give us a vector perpendicular to the plane:
![(0,3,2)*(1,3,0)=\left[\begin{array}{ccc}i&j&k\\0&3&2\\1&3&0\end{array}\right] =(-6,2,-3)](https://img.qammunity.org/2020/formulas/mathematics/college/2zq87q0zs33s9szdf23rx0yu80jzqg48l1.png)
Asuming that the aliens used our conventions, the original plane was perpendicular to the z axis, so that a perpendicular vector to that plane was
(0,0,1)
We know that a dot product between 2 vectors |V.W| = |V| |W| cos(α), where α is the angle between them. If we use the vector perpendicular to this plane, and the one perpendicular to the original plane, α will represent the deviation angle of our new plane.
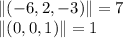

Since this angle is greater than 90 degrees it means that the vector we calculated as perpendicular to the plane points towards negative z (this can be seen by the -3 z component)
To fix this we can calculate a new perpendicular vector, or simply compare ir with the vector (0,0,-1). The latter is easier: