Answer:
11) 6x^2+ 6x^3 = 6.x.x+6.x.x.x
12) -6x^2 + (-6) x^3 = -6.x.x + (-6).x.x.x
13) 5x - 8x = 5.x -8.x
Explanation:
Let us solve the question one by one.
11) 6x^2+ 6x^3
In order to convert the polynomial in the factored form, we see which factors are common in all terms in the polynomial.

The factored form is:
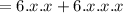
12) -6x^2 + (-6) x^3
Similarly, as 11
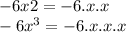
The factored form is:
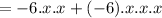
13) 5x - 8x
We can clearly see that there is only one common factor in both terms which is x
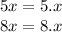
The factored form is:
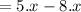
Hence,
11) 6x^2+ 6x^3 = 6.x.x+6.x.x.x
12) -6x^2 + (-6) x^3 = -6.x.x + (-6).x.x.x
13) 5x - 8x = 5.x -8.x