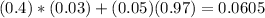Question

In the manufacturing of a chemical adhesive, 3% of all batches have raw materials from two different lots. This occurs when holding tanks are replenished and the remaining portion of a lot is insufficient to fill the tanks. Only 5% of batches with material from a single lot require reprocessing. However, the viscosity of batches consisting of two or more lots of material is more difficult to control, and 40% of such batches require additional processing to achieve the required viscosity. Let A denote the event that a batch is formed from two different lots, and let B denote the event that a lot requires additional processing. Determine the following probabilities:

a. P(A)
b. P(A')
c. P(B\A)
d. P(B\A')
e. P(A ∩ B)
f. P(A ∩ B')
g. P(B)

Answer 1

Explanation:

By the problem we know that our events are:

`A=`A batch is formed from two different lots.

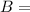`B=`A batch requires additional processing.

So, according to that:

a)
`P(A)=3%=0.03`

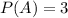
Because P(A) and
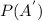`P(A^(') )` are complementary events

b)
`P(A^(') )=1-P(A)=1-0.03=0.97=97%`

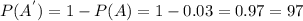
Because of the problem, we know that:

c)
`P(B/A)=0.4=40%`

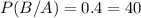
and,

d)
`P(B/A^(') )=0.05=5%`

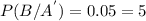
From the formula

e) P(A ∩ B)= P(A)*P(B/A)=
`(0.03)*(0.4)=0.012`

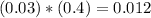
f) P(A ∩ B')=P(A)-P(A ∩ B)=
`0.03-0.012=0.018`

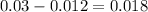
And, finally

g) P(B)=P(B/A)*P(A)+P(B/A')*P(A')=
`(0.4)*(0.03)+(0.05)(0.97)=0.0605`