Question

PLZ DON'T TROLL ACTUALLY ANSWER THE QUESTION CORRECTLY

ALGEBRA 1 QUESTION

Ingrid hit a golf ball. The height of the ball (in meters above the ground) t seconds after being hit is modeled by

h(t)=-5t^2+30t

1) Rewrite the function in a different form (factored or vertex) where the answer appears as a number in the equation.

2) How many seconds after being hit does the ball reach its highest point?

__________seconds

Answer 1

`\huge\boxed{\text{1. } h(t) = -5(t-3)^2 + 45}`

`\huge\boxed{\text{2. 3 seconds}}`

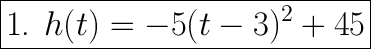
Explanation:

If we're looking for the point at with the ball reaches its highest point, we want to find the graph's vertex. In order to get the equation into a form with the vertex in it, we need to convert the equation into vertex form. This is an equation in the form
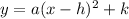`y=a(x-h)^2 + k`, h being the x value of the vertex, and k being the y value of the vertex.

In order to convert our equation,
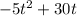`-5t^2+30t` into vertex form, we can algebraically manipulate it to get it into the form we want.

• 
  `-5t^2 +30t`
• 
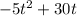
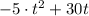  `-5 \cdot t^2 + 30t` (Separate the -5 from the t^2)
• 
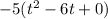  `-5(t^2-6t+0)` (Factor out a -5)
• 
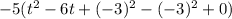  `-5(t^2 -6t + (-3)^2 - (-3)^2 + 0)` (Completing the Square)
• 
  `-5((t-3)^2 - (-3)^2 + 0)` (Binomial Formula)
• 
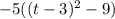  `-5((t-3)^2 - 9)` (Simplify)
• 
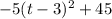  `-5(t-3)^2 + 45` (Factor the -5 back in)

Therefore, our equation with the vertex in it is
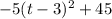`-5(t-3)^2 + 45`. To find the vertex, we can examine the equation to find the values of h and k in the formula
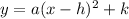`y=a(x-h)^2 + k` (where the vertex coordinates are (h, k)).

It seems that h is 3 and k is 45. Therefore, the coordinate of the vertex is (3, 45). Since it asks for how many seconds after being hit does the ball reach its highest point, we would take the x value since x represents seconds. This is 3. Therefore, the ball reaches its highest point after 3 seconds.

Hope this helped!