Answer:
Yes, x(t)+C is also a solution of given equation.
Explanation:
We are given that x(t) is a solution of the equation x'=f(x)
We have to show that x(t+c) is also a solution of given equation and check x(t)+c is a solution of equation.
Suppose x'=1
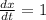
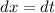
Integrating on both sides
Then , we get
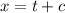
Where C is integration constant.
Now, t replace by t+c
Then, we get
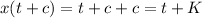 because c+C=K
because c+C=K
Different w.r.t then we get
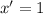
Therefore, x(t+c) is also solution because it satisfied the given equation.
Now, x(t)+C=t+(c+C)=t+L where L=c+C=Constant
Differentiate w.r.t time
Then, we get
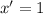
Yes, x(t)+C is also solution of given equation because it satisfied given equation