Question

Nicolet wants to buy a new state of the art computer for R35 000. She decides to save by depositing an amount of R500 once a month into an account earning 11,32% interest per year, compounded monthly. The approximate time it will take Nicolet to have R35 000 available is

Answer 1

54.72 months

Explanation:

Given:

Future value = R35,000

Annuity = R500

Interest = 11.32% per year

Interest per month, r =
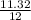`(11.32)/(12)` = 0.943% = 0.00943

Let 'n' be the total time in months taken

Now,

Future value of annuity is calculated using the formula as:

`\textup{Future value}=\textup{Annuity}*[((1+r)^n-1)/(r)]`

on substituting the respective values, we get

`35000=500*[((1+0.00943)^n-1)/(0.00943)]`

or

`70=[((1.00943)^n-1)/(0.00943)]`

or

1.6601 = 1.00943ⁿ

taking log both sides, we get

log(1.6601) = n × log(1.00943)

or

0.22= n × 0.00402

or

n = 54.72 months