Question

Two resistors, 200Ω and 300Ω are in parallel: a) Find their equivalent resistance b) The parallel combination is connected to a 12V battery. Find the current out of the battery c) Find the current flowing in each resistor.

Answer 1

a) 120 Ω

b) 0.1 A

c) 0.06 A , 0.04 A

Step-by-step explanation:

Given:

Resistance R₁ and R₂ = 200 ohms and 300 ohms

Potential difference or voltage = V = 12 V

a) When resistors are in parallel, the equivalent resistance is given by the formula
`(1)/(R)=(1)/(R_(1))+(1)/(R_(2))`

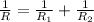
⇒ R =
`[(1)/(R_(1))+(1)/(R_(2))]^(-1)`

=
`[(1)/(200)+(1)/(300)]^(-1)` = (0.00833)⁻¹

⇒ Equivalent resistance = R = 120 ohms.

b) Current out of the battery = I = V/R = 12/120 = 0.1 A.

c) Since the resistors are in parallel, Voltage across each is equal to 12V.

Current flowing in R₁ = V/R₁ = 12 /200 = 0.06 A

Current flowing in R₂ = V/R₂ = 12 /300 = 0.04 A

Answer 2

(A) R =120 ohm (b) 0.1 A (C) current through 200 ohm is 0.06 A and current through 300 ohm is 0.04 A

Step-by-step explanation:

We have two resistances
`R_1=200ohm\ and R_2=300ohm`

(a) As the resistances are connected in parallel

So
`(1)/(R)=(1)/(R_1)+(1)/(R_2)`

`R=(R_1R_2)/(R_1+R_2)=(200* 300)/(200+300)=120ohm`

(b) The combination is connected through 12 V battery

So current
`i=(V)/(R)=(12)/(120)=0.1A`

(C) According to current division rule

Current through 200 ohm resistor
`=0.1* (300)/(200+300)=0.06A`

Now current through 300 ohm resistor = 0.1-0.06=0.04 A